Abstract
Deuterium/Tritium (D/T) handling in defined proportions are pivotal to maintain steady-state operation for fusion reactors. However, the hydrogen isotope effect in metal-hydrogen systems always disturbs precise D/T ratio control. Here, we reveal the dominance of kinetic isotope effect during desorption. To reconcile the thermodynamic stability and isotope effect, we demonstrate a quantitative indicator of Tgap and further a local coordination design strategy that comprises thermodynamic destabilization with vibration enhancement of interstitial isotopes for isotope engineering. Based on theoretical screening analysis, an optimized Ti-Pd co-doped Zr0.8Ti0.2Co0.8Pd0.2 alloy is designed and prepared. Compared to ZrCo alloy, the optimal alloy enables consistent isotope delivery together with a three-fold lower Tgap, a five-fold lower energy barrier difference, a one-third lower isotopic composition deviation during desorption and an over two-fold higher cycling capacity. This work provides insights into the interaction between alloy and hydrogen isotopes, thus opening up feasible approaches to support high-performance fusion reactors.
Introduction
The deuterium-tritium (D-T) fusion reaction that can produce substantial net energy is currently one of the most promising ways to tackle the energy crisis1,2,3,4. However, the development of fusion energy suffers from low fuel burn efficiency, due to the decoupled distributions and distinct transport behaviors of D/T particles5,6,7,8,9. The key to achieve self-sustaining and long-term D-T fusion reaction, thus, lies in fueling with precise D/T ratio. To obtain high-purity and required DT ratio from exhausted fluxes to fuel the plasma, the inner fuel cycle (IFC) of a fusion reactor includes a tokamak exhaust processing system (TEP), an isotope separation system (ISS) and a storage and delivery system (SDS). Owing to the design requirements of a fusion reactor for rapid fueling and accurate isotopic composition, major exhaust gases are processed through the TEP and SDS while the remaining gases are allowed to transfer to the ISS for separation of deuterium and tritium. To this end, the SDS equipped with metal hydride getter beds is designed to achieve the rapid storage of unreacted DT gas and the gas delivery with controllable composition10,11. Nevertheless, the precise control of fuel D/T ratio is always disturbed by the prominent hydrogen isotope effect that originates from the isotopic affinity difference between gas and solid phases12. A list of abbreviations and their corresponding detailed description mentioned in this paper are presented in Supplementary Table 1 to improve the readability.
Separation factor (α)13,14, defined by the ratio of equilibrium isotopic composition in the gas and solid phases (Eq. (1)), is widely used to describe the interphase isotopic distribution difference.
where (Ql/Qh)gas and (Ql/Qh)solid are the atomic fraction of light and heavy isotopes in the gas and solid phases, respectively. Early work shows that the separation factor involving mixed isotopes at certain temperature can be calculated by the ratio of the square root of plateau pressure, determined by Van′t Hoff relationship for single-isotope isotherms (Detailed discussion in Supplementary Note 1). Thus, accurate determination of thermodynamic parameters is crucial for understanding the temperature dependence of isotope effect.
To meet the requirements of SDS, the hydrogen isotope storage candidate prefers ZrCo alloy than depleted uranium (DU) due to the merits of high hydride stability (~10−3 Pa at 20 °C), non-radioactive character, low pyrophoric nature and strong ability of trapping 3He15,16,17,18,19,20,21. Early efforts in ZrCo-based alloys focused mainly on improving the cycling stability through inhibiting the hydrogen-induced disproportionation reaction (HID)15,21,22,23. In recent years, research has expanded to encompass the hydrogen isotope effect of ZrCo-based alloys. Previous researches indicate that the thermodynamic isotope effect of ZrCo alloy exhibits obvious temperature dependence, the plateau pressure for light isotope outweighing that for heavy isotope at a relatively low temperature while the situation could be inverted at an elevated temperature, corresponding to negative and positive isotope effects, respectively. Yet, the reported thermodynamic parameters were scattered (Supplementary Fig. 1)15,16,24,25,26, which would exponentially influence the separation factor and render the accurate thermodynamic isotope effect unattainable.
Moreover, the kinetic isotope effect during rapid storage and delivery processes can be evaluated by thermal analyses and real-time monitoring of gaseous isotopic composition27,28,29,30,31,32. The noticeable fluctuation of isotopic composition during desorption but negligible during absorption with various H/D ratio were observed for ZrCo-based alloys at practical scales, which hinders the stable supply with desirable isotopic ratio27,28,29,30,33. Owing to the prominent kinetic isotope effect, the isotopic composition deviation could be up to 7.30% during temperature programmed desorption with initial H/D = 1/1 in a full-scale ZrCo bed assembly33.
Bridging the gap between the operating temperature (T1 bar) for atmospheric pressure delivery and the critical temperature (Tcr) for zero isotope effect is expected to make impressive gains in delivery performance. It is highly desirable to reduce T1 bar for narrowing the temperature gap and concurrently improving anti-disproportionation ability and cycling stability. On the other hand, the atomic mass distinction among hydrogen isotope atoms results in the zero-point energy (ZPE) difference in both gas and solid phases, dictating the interphase isotopic distribution and further Tcr34,35. More importantly, T1 bar and Tcr show strong sensitivity with the local environment of interstitial isotopes, directly linked to the interstitial coordination atoms. Element substitution, therefore, stands out as a facile strategy for tailoring interstitial coordination environment among modification methods including nanostructuring, surface treatment and mechanical ball-milling36,37,38,39,40,41,42,43,44,45,46,47,48,49,50,51,52,53,54. Although a myriad of efforts have been devoted to reducing the hydrogen isotope effect, the trial-and-error strategy still dominates the alloy development. An effective guiding principle for the alloy design is indispensable.
In this work, the thermodynamic and kinetic isotope effects of ZrCo alloy are precisely obtained. A significant kinetic isotope effect during desorption whereas a negligible one during absorption is observed. The gap (Tgap) between the operating temperature (T1 bar) for atmospheric pressure delivery and the critical temperature (Tcr) for zero isotope effect aggravates the interphase isotopic affinity difference. To alleviate the isotope effect during desorption, a local coordination design strategy striking a balance between thermodynamic stability and isotope effect is established and validated by a series of ZrCo-based alloys. Based on the computational screening results, an optimized Ti-Pd co-doped Zr0.8Ti0.2Co0.8Pd0.2 alloy is designed, prepared, and compared with ZrCo, Zr0.8Ti0.2Co and ZrCo0.8Pd0.2 alloys. Ti-Pd co-doping enables further suppressed isotope effect than Ti and Pd single doping. Specifically, the Tgap of Zr0.8Ti0.2Co0.8Pd0.2 alloy reaches 83.99 °C while that of ZrCo, Zr0.8Ti0.2Co and ZrCo0.8Pd0.2 alloys are 251.62, 172.00 and 197.41 °C, respectively. Moreover, Zr0.8Ti0.2Co0.8Pd0.2 alloy exhibits enhanced cycling stability and a reduction of 30.18% in the maximum of isotopic composition fluctuation during desorption compared to pristine ZrCo alloy. This proposed strategy provides fundamental insights into the hydrogen isotope effect in metal-hydrogen systems and prompts employment of ZrCo-based alloys getter bed for advanced nuclear fusion.
Results
Characterization and hydrogen isotope effect of ZrCo alloy
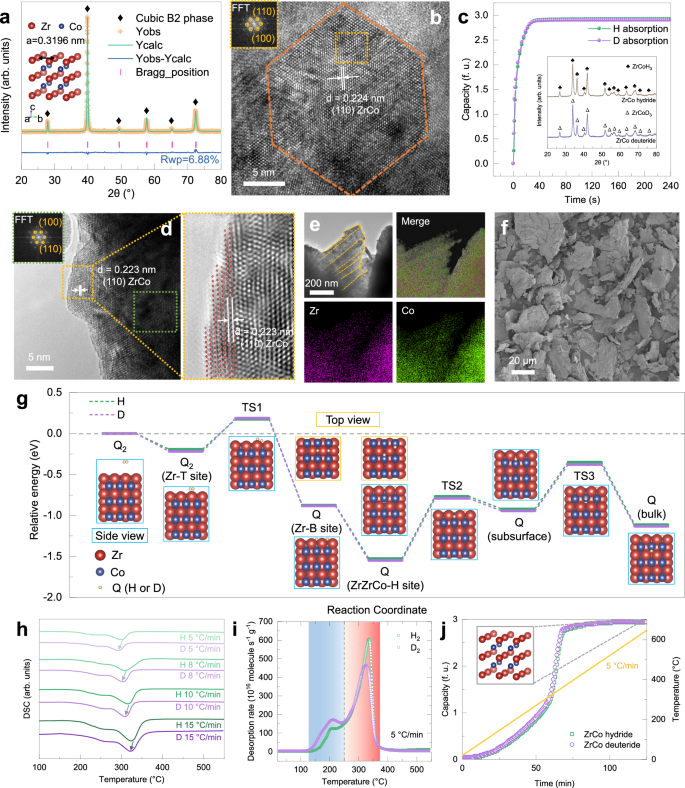
The X-ray diffraction (XRD) refinement and high resolution transmission electron microscopy (HRTEM) verify that the ZrCo alloy with single cubic B2 phase was successfully fabricated for determining accurate isotope effect (Fig. 1a, b). The hydrogen isotope absorption kinetics under atmospheric pressure at room temperature in single- and mixed-isotope systems exhibit no distinct difference in both absorption capacity and rate (Fig. 1c). Specifically, the absorption capacity is near the theoretical capacity (~3 f.u.) and the absorption time until 90% capacity is less than 60 s for both protium and deuterium. Such negligible kinetic isotope effect for absorption benefits rapid isotope storage, resulting from two aspects: (i) the significant driving force originating from the system pressure (~105 Pa) far beyond the equilibrium plateau pressure at room temperature (~10−3 Pa); (ii) the close-packed crystal plane (110) with preferential orientation in the cubic B2 phase, validated by the highest diffraction intensity and dominance of (110) plane in the grain and surface (Fig. 1a, b, d). Moreover, the layered morphology with chemical homogeneity over interlayer and bulk regions was observed (Fig. 1d–f). Generally, an absorption process proceeds, in the metal-hydrogen system, through adsorption, dissociation and diffusion processes. The NEB calculation with zero point energy (ZPE) correction based on the experimental observation and theoretical prediction55 was thus conducted to elucidate the kinetic isotope effect during absorption (Fig. 1g and Supplementary Table 2). The energy barriers from surface to subsurface and further bulk are 0.76 and 0.57 eV, respectively, which is consistent with previously reported results55,56. The energy difference between ZrCo-H/D systems at each step during absorption is less than 0.04 eV, which suggests a slight kinetic isotope effect for absorption. This contributes to further investigation of the kinetic isotope effect for desorption, which means that one can control the isotopic ratio in the solid phase by that in the gas phase.
a XRD pattern and Rietveld refinement result of ZrCo alloy. b HRTEM image of a ZrCo grain with the inset graph displaying the FFT image of the region of interest (orange dashed line). c Absorption kinetic curves with protium and deuterium, respectively. Inset image shows the XRD patterns of ZrCo hydride and deuteride. d HRTEM image of ZrCo particles on the surface. e Bright field and corresponding STEM-HAADF image with EDS. f SEM image of ZrCo particles. g Energy variation of ZrCo-Q (Q = H, D) systems during adsorption, dissociation and diffusion processes with ZrCo (110) plane. T, B and H sites represent top, bridge and hollow sites, respectively. h DSC curves of ZrCo hydride and deuteride with a series of heating rates (5, 8, 10, 15 °C/min). i TDS curves of ZrCo hydride and deuteride with the heating rate of 5 °C/min. j TPD profiles of ZrCo hydride and deuteride with the heating rate of 5 °C/min. In this article, ‘f. u.’ is adopted as the unit of capacity, which means the mole ratio of isotope and alloy, for isotopic distinction.
Differential scanning calorimetry (DSC), temperature programmed desorption (TPD) and thermal desorption spectroscopy (TDS) were used to evaluate the kinetic isotope effect for desorption in single-isotope systems. The presence of a main desorption peak with a shoulder in the DSC and TDS is attributed to the reaction of ZrCoH3 → ZrCo with solute hydrogen release, supported by the XRD patterns at different temperatures (Fig. 1h, i, Supplementary Fig. 2). Additionally, ZrCo deuteride exhibits a lower peak temperature than its hydride in the DSC and TDS, indicating the faster desorption kinetics and weaker thermodynamic stability of deuteride, which accords with the TPD results (Fig. 1j). We further give a quantitative description of the kinetic isotope effect for desorption based on the Kissinger relationship between
and
(Eq. (2)). Both the fitting plots depict an obvious linear correlation, of which the slope represents the apparent activation energy (Ea) for desorption (Supplementary Fig. 3). The Ea of ZrCo hydride/deuteride are 111.42 and 97.29 kJ/mol, respectively. The substantial difference between isotopic energy barriers leads to a significant kinetic isotope effect for desorption and limits accurate isotope supply.
where β is the heating rate, Tp represents the peak temperature of DSC curve and A signifies the pre-exponential factor.
To illustrate the difference of thermodynamic stability between hydride and deuteride over a wide temperature range, a series of pressure-composition isotherms (PCIs) were measured (Fig. 2a and Supplementary Fig. 4), of which the plateau pressure (Peq) is reckoned as an indicator for thermodynamic stability. The single plateau region is attributed to the one-step reaction, i.e., 2ZrCo + 3Q2 ↔ 2ZrCoQ3 (Q = H, D), which is in accordance with the DSC and TDS analysis. The temperature dependence of thermodynamic stability was described by Van′t Hoff relationship between lnPeq and T−1 (Fig. 2b), which manifests a strong linear correlation (R2 > 99.9%) and thus a good reliability. Specifically, the isotopic thermodynamic parameters of ZrCo alloy were summarized in Table 1.
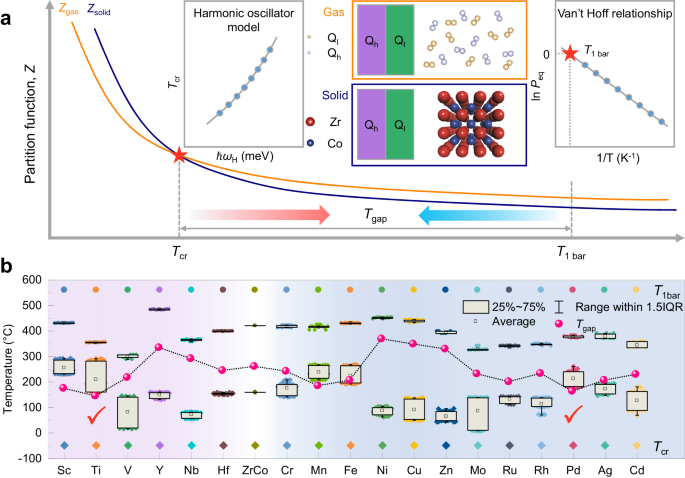
a PCIs of ZrCo alloy for protium absorption and desorption which are represented by open and solid legends, respectively. b Van′t Hoff plot of ZrCo alloy for protium/deuterium absorption and desorption. c Schematic illustration of the potential well curves for molecular and interstitial isotopes. d Dependence of separation factor α on the temperature and interstitial vibration energy ћωH. e Relationship between the critical temperature Tcr and interstitial vibration energy ћωH for binary isotope mixture.
Indicator for the mismatch between thermodynamic stability and isotope effect
It is worth noting that the disparity between the temperature dependences of the thermodynamic stability of ZrCo hydride and deuteride owing to the isotopic differences of enthalpy and entropy is observed (Fig. 2c). The entropy change (ΔS) operates in the opposite direction compared with the enthalpy change (ΔH) for thermodynamic stability. Moreover, its contribution can be magnified with temperature, leading to the inversion of isotope effect (Fig. 2b), which was also reported in the conventional LaNi5-Q2 (Q = H, D) systems57. Similar isotopic inversion phenomena were observed in the low-dimensional materials58,59. Herein, an evaluation indicator (critical temperature (Tcr)), at which hydride and deuteride share the same thermodynamic stability, could be thus readily defined as
The Tcr for desorption was extrapolated to be 157.11 °C and corresponding plateau pressure is 14 Pa, which is too low to be experimentally evidenced. Inspiringly, the reversible character and large hysteresis between absorption and desorption processes induced by the intrinsic structure-change phase transition allow direct observation of such inversion phenomenon in the absorption process. The difference between plateau pressures for H/D absorption at 150 and 175 °C clearly shows the inversion of thermodynamic isotope effect (Fig. 2b). The accurate Tcr for absorption was further calculated to be 161.12 °C, which is very close to the value for desorption. The reversibility of absorption and desorption reactions provides us an effective alternative for experimentally determining isotope effect for forward and reverse reactions.
Delivery at the Tcr can enable alleviated isotopic composition fluctuation in favor of the steady-state operation of plasma burning. Not only the isotope effect but also the delivery pressure should be considered for practical application. To this end, another evaluation indicator (operating temperature (T1 bar)) (Eq. (4)), which represents the temperature for atmospheric pressure delivery, was introduced to characterize the thermodynamic stability of hydride phase.
In light of the large hysteresis of ZrCo alloy, a relatively high T1 bar is required (>400 °C), far beyond the corresponding Tcr (157.11 °C). In such case, the contribution to thermodynamic stability of entropy change (ΔS) would outweigh that of enthalpy change (ΔH), which leads to the heavy isotope-rich gas during delivery. Furthermore, a comprehensive indicator (temperature gap (Tgap)), integrating the isotope effect with thermodynamic stability of metal hydride for isotope storage and delivery was proposed (Eq. (5)).
The mismatch between T1 bar and Tcr challenges the compatibility of ZrCo alloy as an isotope storage and delivery material with other components in the tritium plant. The reduction of Tgap is expected to mitigate the isotope effect during desorption and improve the composition accuracy of isotope supply.
Establishment of local coordination design strategy
As a theoretical model referring to the hydrogen atom behavior in the metal-hydrogen system34,35, the harmonic oscillator model applies the partition functions of molecular and interstitial hydrogen isotopes to deduce the separation factor (Detailed discussion in Supplementary Note 2).
where the Zsolid and Zgas represent the partition function for hydrogen isotopes in the gas and solid phases, respectively. It is worth noting that the temperature dependence of separation factor is vulnerable to the interstitial vibration energy (ℏωH) (Fig. 2d). In detail, Tcr shows a positive correlation with ℏωH, which depends weakly on the pair of isotope mixture (H-D and H-T pairs) (Fig. 2e). This means that the conclusion in the H-D mixed system is hopeful to be extended to the tritium-related system.
Both the elevated Tcr and decreased T1 bar contribute to a narrowed Tgap (Fig. 3a). Essentially, the thermodynamic stability and vibration energy of interstices were defined by the coordinated atoms around interstitial hydrogen isotope in the lattice. Herein, the Zr0.75A0.25Co (A=Sc, Ti, V, Y, Zr, Nb, Hf) for Zr site doping and the ZrCo0.75B0.25 (B=Cr, Mn, Fe, Co, Ni, Cu, Zn, Mo, Ru, Rh, Pd, Ag, Cd) for Co site doping were constructed and optimized to screen out the most favorable element for diminishing Tgap (Fig. 3b and Supplementary Table 3). The decreasing order of T1 bar follows the decreasing order of doping atom size, which reproduces well the Lundin theory60. In addition, the obvious staged distribution of Tcr reflects the sensitivity of vibration energy to the local coordination environment of interstitial hydrogen. Ti and Pd were reckoned as the optimal elements to minimize Tgap based on the computational screening results. Considering the excellent anti-disproportionation performance, a promising Zr0.8Ti0.2Co alloy was prepared firstly for further investigation.
Contribution of Ti single doping in the hydrogen isotope absorption and desorption behaviors for Zr0.8Ti0.2Co alloy
The as-cast Zr0.8Ti0.2Co alloy with single cubic B2 phase was prepared (Fig. 4a). In detail, the right shift of diffraction peaks suggests the decreased lattice parameters compared with pristine ZrCo alloy. Moreover, the EDS mapping results reveal the chemically homogeneous nature of Zr0.8Ti0.2Co alloy (Fig. 4b). Additionally, the TEM and HRTEM images give a detailed microstructure information that (110) plane, the close-packed crystal plane of cubic B2 phase, is preferentially presented (Fig. 4c, d). This infers a minor isotope effect during absorption that is supported by the insignificant difference between the diffraction peaks of Zr0.8Ti0.2Co hydride and deuteride (Fig. 4e). To simulate the isotope storage process with the stoichiometry of deuterium and tritium required by the fusion reaction (Eq. (7)), the absorption measurement with equimolar isotope mixture was conducted (Fig. 4f). Furthermore, the protium content in the gas phase during absorption was monitored by gas chromatography. These results demonstrate that Zr0.8Ti0.2Co alloy has a fast absorption kinetics with a negligible isotope effect comparable as pristine ZrCo alloy.
a XRD pattern and Rietveld refinement result, b,c TEM and HRTEM images, d HAADF-STEM image with EDS of Zr0.8Ti0.2Co alloy. e XRD patterns of Zr0.8Ti0.2Co hydride and deuteride. f Hydrogen concentration variation in the gas phase during absorption with H/D = 1/1 mixed hydrogen isotopes. g PCIs of Zr0.8Ti0.2Co alloy for protium absorption and desorption which are represented by open and solid legends, respectively. h Kissinger fitting curves for desorption of ZrCo and Zr0.8Ti0.2Co hydride/deuteride, respectively. i TDS curve of Zr0.8Ti0.2Co hydride. j Tgap of ZrCo and Zr0.8Ti0.2Co alloys.
PCI and related phase component analysis (Fig. 4g, Supplementary Figs. 5, 6) provide direct evidence of the decisive effect of Ti doping on the thermodynamic behavior for Zr0.8Ti0.2Co alloy: (i) dual plateau for absorption while single plateau for desorption, i.e., absorption-desorption asymmetry, was observed. In addition, the significant difference among Tcr for absorption and desorption also suggests disparate reaction pathways; (ii) thermodynamic destabilization of interstitial isotope atoms was realized. In detail, the thermodynamic parameters were calculated by Van′t Hoff fitting, as displayed in Table 2. The lower enthalpy change for desorption of Zr0.8Ti0.2Co hydride/deuteride compared with ZrCo hydride/deuteride boosts isotope delivery ability and contributes to a decreased Tgap.
To elucidate the kinetic pathway that is intimately related to the establishment of thermodynamic equilibrium state, DSC curves were obtained as the desorption kinetics of Zr0.8Ti0.2Co hydride/deuteride. The DSC plots exhibit two endothermic peaks including a weak peak with a relatively low peak temperature and a main peak during desorption (Supplementary Fig. 7). Owing to the size effect60, Ti single doping enables Zr0.8Ti0.2Co alloy with a significantly decreased main peak temperature for desorption. Moreover, the reduced slope value of the Kissinger plots for Zr0.8Ti0.2Co alloy indicates a decreased Ea for desorption, which agrees well with the thermodynamic conclusions (Fig. 4h). The Ea of hydride and deuteride are reduced from 111.42 and 97.29 kJ/mol for ZrCo alloy to 77.96 and 68.65 kJ/mol for Zr0.8Ti0.2Co alloy, respectively. The reduced difference between energy barriers helps to mitigate the isotope effect during desorption. To clarify the reason for the asymmetry of absorption and desorption processes, the TDS of Zr0.8Ti0.2Co hydride was measured (Fig. 4i). As per the intensity evolution of gas species in the TDS and the XRD patterns at different temperatures (Supplementary Fig. 8), the desorption process can be divided into three steps: (i) hydrogen release within β phase region; (ii) phase transformation from orthorhombic β phase to the intermediate hydride phase with cubic structure (B2′ phase); (iii) isostructural phase transition with cubic structure from intermediate hydride phase to cubic B2 phase. The overlap between step (ii) and step (iii) confirms simultaneous hydrogen release corresponding to the phase transformations of β → B2′ and B2′ → B2, further leading to the thermodynamically unstable state for B2′ phase during desorption.
Although the Tgap decreased from 251.62 °C for ZrCo alloy to 172.00 °C for Zr0.8Ti0.2Co alloy (Fig. 4j), the reduction is mainly attributed to the decreased T1 bar, associated with the similar electronic configuration and decreased atomic size of Ti compared with Zr. Hence, the interstitial size effect dominates the way for the reduction of Tgap, but is slightly effective for the improvement of Tcr. Considering the lowest simulated Tgap for Pd among Co site substitution elements and profound isotope effect of Pd metal61,62,63,64, a Ti-Pd co-doped Zr0.8Ti0.2Co0.8Pd0.2 alloy was rationally designed to further narrow the Tgap.
Synergistic effect of Ti-Pd co-doping on hydrogen isotope effect and cycling stability for Zr0.8Ti0.2Co0.8Pd0.2 alloy
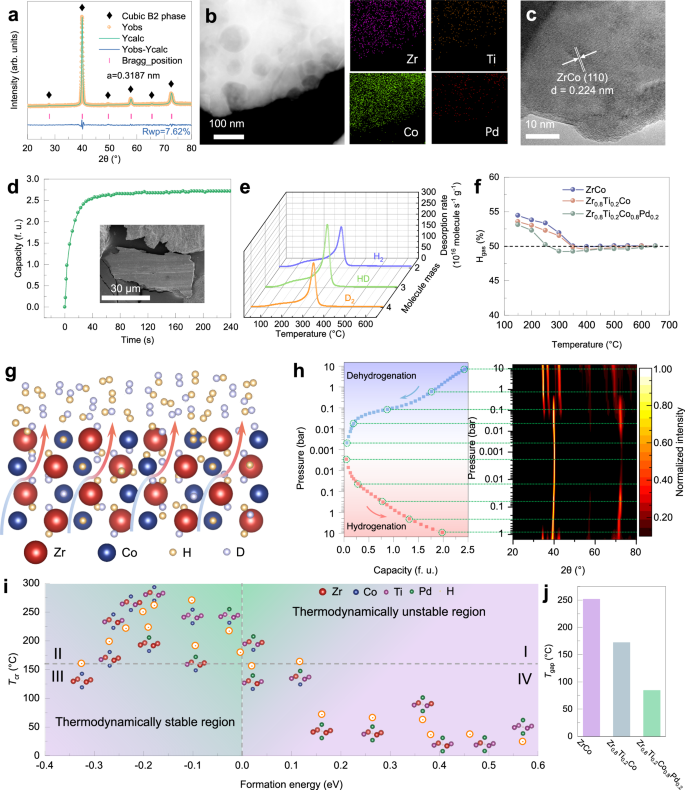
Zr0.8Ti0.2Co0.8Pd0.2 alloy maintains single cubic B2 phase with homogenous element distribution (Fig. 5a, b). The (110) preferential orientation can also be observed by HRTEM (Fig. 5c). Therefore, the layered alloy intends to keep favorable absorption kinetics with H2/D2 = 1/1 gas mixture at room temperature as ZrCo and Zr0.8Ti0.2Co alloys (Fig. 5d, Supplementary Fig. 9). The Kissinger fitting results in single-isotope systems indicate further reduction of the energy barrier difference between hydride (93.74 kJ/mol) and deuteride (90.81 kJ/mol), which contributes to a mitigated isotope effect (Supplementary Fig. 10). Additionally, the significant kinetic improvement resulting from Ti-Pd co-doping is verified by TPD measurements for ZrCo, Zr0.8Ti0.2Co and Zr0.8Ti0.2Co0.8Pd0.2 hydrides (Supplementary Fig. 11), which ensures high fuel process fluxes. To explore the kinetic isotope effect during desorption in mixed-isotope systems, the TDS profiles of ZrCo, Zr0.8Ti0.2Co and Zr0.8Ti0.2Co0.8Pd0.2 alloys saturated in the gas mixture of H2/D2 = 1/1 were obtained with the heating rate of 5 °C/min. As is evident in Fig. 5e and Supplementary Figs. 12, 13, three gas species including H2, HD and D2 were all detected during the release process. The consistent onset and peak temperatures among all three gas species signify barely preferential release of any isotopologue. Moreover, HD dominates the TDS among all three species. To quantitatively track the isotopic composition during the whole desorption process, the intensity of H2, HD and D2 species were calibrated. Especially, the HD calibration can be achieved based on the law of hydrogen isotope mass conservation and negligible hydrogen isotope effect of ZrCo-based alloys during absorption. The temperature dependence of the hydrogen concentration of accumulative release gas during desorption was determined. The variation amplitude of isotopic composition in the release gas becomes gradually flat from pristine ZrCo alloy to Zr0.8Ti0.2Co alloy and further Zr0.8Ti0.2Co0.8Pd0.2 alloy (Fig. 5f), which reflects the substantial suppression of isotope separation and significant improvement for stable isotope storage and delivery. The detailed data at certain temperatures are list in Supplementary Table 4. Specifically, Ti-Pd co-doping renders a reduction of 30.18% in the maximum of isotopic composition fluctuation during desorption compared with ZrCo alloy, which validates the design protocol. Figure 5g gives a schematic illustration for the desorption process of the hydride phase with mixed hydrogen isotopes. Initially, isotope atoms randomly locate in the lattice interstices of hydride phase. With temperature increasing, they are excited to diffuse from bulk to subsurface and further surface. Combination of isotope atoms occurs on the surface and the molecules with different isotopic modifications are then released into the gas phase65. The subsequent isotope exchange process is involved with the interphase isotope exchange between gas and solid phases and homomolecular isotope exchange reaction (HMIE) in the gas phase. In addition, Zr0.8Ti0.2Co0.8Pd0.2 alloy shows a discrepancy of thermodynamic pathway between absorption and desorption processes, which is also observed in Zr0.8Ti0.2Co alloy (Fig. 5h). The unapparent plateau region for absorption makes the reaction path elusive. Herein, a series of XRD patterns with different hydrogen contents reveals a two-step reaction mechanism for absorption: (i) formation of the intermediate hydride phase with cubic structure (B2′ phase); (ii) structure-change phase transformation from cubic B2′ phase to orthorhombic ZrCoH3 phase. As for desorption, the single plateau region corresponding to the reaction of orthorhombic ZrCoH3 phase to cubic B2 phase is reconfirmed. Based on the PCIs (Supplementary Figs. 14–17), the corresponding thermodynamic parameters of Zr0.8Ti0.2Co0.8Pd0.2 alloy are listed in Supplementary Table 5 and Table 3. Furthermore, the salient driving force caused by the substantial gap between system pressure and equilibrium plateau pressure at room temperature (0.136 Pa at 20 °C) that fitted from Van’t Hoff equation ensures the rapid storage kinetics and thus tiny kinetic isotope effect during absorption. For desorption, the minor difference of T1 bar between Zr0.8Ti0.2Co0.8Pd0.2 and Zr0.8Ti0.2Co alloys specifies the little influence of Pd substitution for Co on the stability of hydride phase. It should be noted that the mitigated Tgap is mainly attributed to the increase of Tcr.
a XRD pattern and Rietveld refinement result, (b) HRTEM image, (c) HAADF-STEM image with EDS of Zr0.8Ti0.2Co0.8Pd0.2 alloy. d Typical hydrogenation kinetic behavior of Zr0.8Ti0.2Co0.8Pd0.2 alloy with layered microstructure which can be observed from the inset SEM image. e TDS of Zr0.8Ti0.2Co0.8Pd0.2 sample saturated in the mixed gas with H2/D2 = 1/1. f Cumulative hydrogen concentration of release gas during the whole desorption process. g Schematic illustration of the desorption process of the hydride phase with mixed hydrogen isotopes. h Phase identification along the de-/hydrogenation processes of Zr0.8Ti0.2Co0.8Pd0.2 alloy. i Correlation between Tcr and corresponding formation energy of interstitial isotopes in Zr0.8Ti0.2Co0.8Pd0.2 alloy. j Tgap of ZrCo, Zr0.8Ti0.2Co and Zr0.8Ti0.2Co0.8Pd0.2 alloys.
To understand the explicit contribution of Pd single doping for hydrogen isotope effect, ZrCo0.8Pd0.2 alloy was prepared. The single B2 phase, hierarchical layered microstructure at the micro/nanometer scales and preferred orientation of (110) plane are also demonstrated by XRD, SEM and TEM (Supplementary Figs. 18 and 19a, b). Additionally, the STEM-HAADF image and corresponding EDS mapping demonstrate the homogeneous distribution and accurate content of alloy elements (Supplementary Fig. 19c, d). The thermodynamic parameters of ZrCo0.8Pd0.2 alloy were obtained based on a series of PCIs (Supplementary Figs. 20–23) and listed in Supplementary Table 6. The single plateau region and nearly consistent Tcr for both absorption and desorption processes confirm the reversibility of phase transition and the reliability to deduce isotopic behaviors from reversible reactions. The Tgap for ZrCo0.8Pd0.2 alloy is 197.41 °C, of which the reduction is mainly contributed to the increase of Tcr from 157.11 °C for ZrCo alloy to 193.31 °C for ZrCo0.8Pd0.2 alloy.
To elaborate the interaction between the interstitial hydrogen atom and Zr0.8Ti0.2Co0.8Pd0.2 alloy matrix, all possible interstices were constructed and optimized. According to the harmonic oscillator model and density functional theory (DFT) calculations, there is a close correlation between the formation energy and Tcr for interstitial hydrogen atoms (Fig. 5i). All interstices with negative formation energy exhibit improved Tcr (Zone II) whereas those with positive formation energy are expected to have decreased Tcr (Zone IV). This means all thermodynamically stable interstices can assist in enhancing the Tcr of Zr0.8Ti0.2Co0.8Pd0.2 alloy. More importantly, Ti-Pd co-doping synergistically induces vibration enhancement, i.e., increased Tcr, as well as thermodynamic destabilization, i.e., decreased T1 bar, within the thermodynamically stable zone (Zone II), which remarkably narrowed the Tgap from 251.62 °C (ZrCo alloy) to 83.99 °C (Zr0.8Ti0.2Co0.8Pd0.2 alloy) (Fig. 5j).
Furthermore, the cycling stability of isotope storage and delivery materials proves critical for applicability in the tritium-related field. Targeting the tritium self-sufficiency and operational safety for fusion reactors, the design of tritium plant should minimize the tritium start-up inventory and maintain the limited reserve tritium inventory. The SDS is required to provide stable cycling processes to meet these stringent requirements. Thus, we further evaluated the effect of Ti-Pd co-doping on the cycling stability of Zr0.8Ti0.2Co0.8Pd0.2 alloy with deuterium, where the specific process parameters are shown in Supplementary Fig. 24.
As demonstrated in Supplementary Fig. 25, the capacity retention and stable cyclic capacity of Zr0.8Ti0.2Co0.8Pd0.2 alloy after 50 cycles are 61.94% and 1.66 f. u., over twofold higher than 25.91% and 0.715 f. u. of ZrCo alloy, which emphasizes the cooperative enhancement of Ti-Pd co-doping to retard disproportionation and prolong cycling stability by thermodynamic destabilization. The cycling capacity attenuation of Zr0.8Ti0.2Co0.8Pd0.2 alloy arises from two aspects: (i) the hydrogen-induced disproportionation reaction (HID); (ii) the isostructural phase transition with cubic structure induced by spatial confinement of in situ disproportionation products (ZrCo2 and ZrH2). The contradictory results between appreciable capacity fading and trace amount of disproportionation products in the XRD profiles (Supplementary Fig. 25c, d) confirm the much larger contribution of the latter to capacity attenuation, which is favorable to the minimization of tritium inventory and retention.
In summary, through comprehensive experimental and theoretical analysis, we obtain accurate isotopic performance and elucidate corresponding mechanism for ZrCo alloy. To alleviate the isotopic composition deviation governed by the kinetic isotope effect during desorption, we propose a quantitative indicator of Tgap to describe the mismatch between thermodynamic stability and isotope effect, and further establish a local coordination design strategy coupling thermodynamic destabilization with vibration enhancement of interstitial isotopes for rational isotope engineering. A minimized Tgap is desired to realize precise isotope supply to ensure safe and efficient operation of plasma burning. Based on the computational screening results, Ti and Pd elements are screened out, and an optimized Ti-Pd co-doped Zr0.8Ti0.2Co0.8Pd0.2 alloy is designed and prepared. Ti-Pd co-doping endows Zr0.8Ti0.2Co0.8Pd0.2 alloy a one-third lower isotopic composition deviation during desorption and an over two-fold higher cycling capacity compared with pristine ZrCo alloy. The explicit contribution of Ti/Pd single doping and synergistic effect of Ti–Pd co-doping on the hydrogen isotope effect are elaborated. The impressive success in suppressing hydrogen isotope effect validates the design principle for the isotope engineering of ZrCo-based alloys, thus prompting the advancement of isotope storage and delivery material in nuclear fusion.
Methods
Sample preparation
Zirconium (99.9%) and cobalt (99.9%) were supplied by General Research Institute for Non-ferrous Metals. Titanium (99.9%) was purchased from Alfa Aesar. Palladium (99.95%) was purchased from Beijing Dream Material Technology Co., Ltd. As a typical synthesis of alloy, ZrCo, Zr0.8Ti0.2Co, ZrCo0.8Pd0.2 and Zr0.8Ti0.2Co0.8Pd0.2 alloys were prepared by induction levitation melting method. High purity metals based on the chemical composition were melted together followed by remelting process for four times in a water-cooled copper crucible to ensure chemical homogeneity. During melting process, the melting chamber was full of argon atmosphere of 1.4 bar to protect the sample from oxidation.
Characterizations
X-ray diffraction (XRD) patterns were obtained on an X-ray powder diffractometer (Smartlab, Rigaku) with Cu Kα radiation (λ = 1.5406 Å). FullProf Suite program was employed to determine lattice constants by Rietveld refinement. The microstructure was observed by scanning electron microscopy (SEM, Hitachi SU-70) and transmission electron microscopy (TEM, Tecnai G2 F20 S-TWIN) equipped with energy dispersive X-ray spectrometer (EDS, Oxford X-MAX 80 T).
Hydrogen isotope effect measurements
The as-cast samples were activated at 500 °C under vacuum condition after being polished by grinding wheel. Further, 5 bar pure hydrogen was loaded at 100 °C and maintained for several hours until the activation process was completed. The activated samples were preserved in an argon-filled glove box with O2 and H2O level less than 0.01 ppm for subsequent experiments. The PCIs (Pressure-composition isotherms) were obtained by a series of gas-solid equilibrium under different hydrogen pressures at a setting temperature in the self-made Sievert′s type platform (leak rate<1 × 10−9 cc/sec, Agilent). The hydrogen pressure was measured by vacuum gauges (1 Torr and 1000 Torr, INFICON) and pressure transmitter (10 bar ABS, WIKA). Hydrogen isotope absorption/desorption kinetics were recorded by a VGC503 (INFICON). The gas chromatograph (GC, Agilent 7890B) equipped with a molecular sieve column (5 A) and thermal conductivity detector (TCD) was employed to monitor the gas composition during absorption. Differential scanning calorimetry (DSC) was conducted on a simultaneous thermal analyzer (NETZSCH, STA449 F3 Jupiter) from 20 to 550 °C. Temperature programmed desorption (TPD) curves were obtained from room temperature to setting temperature with the heating rate of 5 °C/min. Thermal desorption spectroscopy (TDS) using a quadrupole mass spectrometer (QMS) was adopted to evaluate isotopic selectivity from 20 to 650 °C with the heating rate of 5 °C/min. Sample powder with certain isotopic composition was placed in the chamber and pre-evacuated by turbomolecular pump. The desorption signals corresponding to isotopically pure gas components were calibrated by standard gases. Absorption and desorption cycle tests consist of deuterium absorption under atmospheric pressure at room temperature and temperature programmed desorption with deuterium from room temperature to 380 °C.
Theoretical calculations
The first-principle calculations were conducted using density functional theory (DFT) based on the Vienna Ab Initio Simulation Package (VASP). The electronic exchange-correlation interaction was described by the projected augmented wave (PAW) method and generalized gradient approximation (GGA) together with functional of Perdew–Burke–Ernzerhof (PBE). A kinetic energy cutoff of 400 eV was applied for theoretical simulations. The convergence criterion and ionic relaxation criterion were set to 1 × 10−6 eV and 0.01 eV Å−1, respectively.
For the simulation of isotope absorption process, the 3 × 2 supercell with ZrCo (110) plane containing a slab of six atomic layers (top three relaxed, bottom three fixed) and 15 Å vertical vacuum space was established. The climbing image nudging elastic band (CI-NEB) method was employed to track the energy variation during adsorption, dissociation and diffusion processes for both protium and deuterium. The zero-point energy (ZPE) correction was carried out to determine the formation energy and energy barrier.
For doping elements screening, Zr0.75A0.25Co (A=Sc, Ti, V, Y, Zr, Nb, Hf) for Zr site doping and ZrCo0.75B0.25 (B=Cr, Mn, Fe, Co, Ni, Cu, Zn, Mo, Ru, Rh, Pd, Ag, Cd) for Co site doping with supercell of 2 × 2 × 2 for cubic phase were constructed. A k-point mesh of 4 × 4 × 4 was used for Brillouin zone sampling. The calculation of vibration frequency for interstitial isotope was conducted with the ground state structures after geometry optimization. For exploring the vibration energy of interstitial isotope for all possible interstices in Zr0.8Ti0.2Co0.8Pd0.2 alloy, supercell of 3 × 3 × 3 for cubic phase was employed. The k-point meshes of 4 × 4 × 4 was used for Brillouin zone sampling. Crystal structures were built using Visualization for Electronic and Structure Analysis (VESTA) software.
Data availability
The source data that support the findings of this study are available from the corresponding author upon request.
Code availability
The codes that support the findings of this study are available from the corresponding author upon request.
References
-
Kritcher, A. L. et al. Design of inertial fusion implosions reaching the burning plasma regime. Nat. Phys. 18, 251–258 (2022).
-
Kembleton, R. & Bustreo, C. Prospective research and development for fusion commercialisation. Fusion Eng. Des. 178, 113069 (2022).
-
Sizyuk, V. & Hassanein, A. New proposed ITER divertor design using carbon insert on tungsten to mitigate ELMs and secondary radiation effects on nearby components. Sci. Rep. 12, 4698 (2022).
-
Le Pape, S. et al. Fusion energy output greater than the kinetic energy of an imploding shell at the National Ignition Facility. Phys. Rev. Lett. 120, 245003 (2018).
-
Bucalossi, J. et al. Operating a full tungsten actively cooled tokamak: overview of WEST first phase of operation. Nucl. Fusion 62, 042007 (2022).
-
Hoelzl, M. et al. The JOREK non-linear extended MHD code and applications to large-scale instabilities and their control in magnetically confined fusion plasmas. Nucl. Fusion 61, 065001 (2021).
-
Mailloux, J. et al. Overview of JET results for optimising ITER operation. Nucl. Fusion 62, 042026 (2022).
-
Zhu, D. et al. In situ melting phenomena on W plasma-facing components for lower divertor during long-pulse plasma operations in EAST. Nucl. Fusion 63, 036022 (2023).
-
Juarez, R. et al. A full and heterogeneous model of the ITER tokamak for comprehensive nuclear analyses. Nat. Energy 6, 150–157 (2021).
-
Kenjo, S. et al. Employing of ZrCo as a fuel source in a discharge-type fusion neutron source operated in self-sufficient mode. Int. J. Hydrog. Energy 47, 3054–3062 (2022).
-
Ananyev, S. S. et al. Concept development and candidate technologies selection for the DEMO-FNS fuel cycle systems. Nucl. Fusion 61, 116062 (2021).
-
Hao, S. & Sholl, D. S. Using first-principles calculations to accelerate materials discovery for hydrogen purification membranes by modeling amorphous metals. Energy Environ. Sci. 1, 175–183 (2008).
-
Lozada-Hidalgo, M. et al. Sieving hydrogen isotopes through two-dimensional crystals. Science 351, 68–70 (2016).
-
Anand, N. S., Pati, S., Jat, R. A., Parida, S. C. & Mukerjee, S. K. Hydrogen isotope effect on thermodynamic properties of Pd0.9X0.1 (X = Cu, Ag and Au) alloys. Int. J. Hydrog. Energy 42, 3136–3141 (2017).
-
Bekris, N., Besserer, U., Sirch, M. & Penzhorn, R. D. On the thermal stability of the zirconium/cobalt–hydrogen system. Fusion Eng. Des. 49-50, 781–789 (2000).
-
Yao, Z. et al. An in-depth study on the thermodynamics and kinetics of disproportionation behaviors in ZrCo-H system. J. Mater. Chem. A 8, 9322–9330 (2020).
-
Kou, H. et al. Experimental study on full-scale ZrCo and depleted uranium beds applied for fast recovery and delivery of hydrogen isotopes. Appl. Energy 145, 27–35 (2015).
-
Ye, R. et al. Hydrogen storage and heat transfer properties for large-capacity double thin-layered annulus ZrCo bed with secondary containment cavity. Int. J. Hydrog. Energy 47, 8446–8456 (2022).
-
Shim, M. et al. Initial test results of a fast heat transfer response ZrCo hydride bed. Fusion Sci. Technol. 56, 856–860 (2009).
-
Hayashi, T., Suzuki, T. & Okuno, K. Long-term measurement of He-3 release behavior from zirconium-cobalt tritide. J. Nucl. Mater. 212, 1431–1435 (1994).
-
Liang, Z., Xiao, X., Qi, J., Kou, H. & Chen, L. ZrCo-based hydrogen isotopes storage alloys: a review. J. Alloy. Compd. 932, 167552 (2023).
-
Bekris, N. & Sirch, M. On the mechanism of the disproportionation of ZrCo hydrides. Fusion Sci. Technol. 62, 50–55 (2012).
-
Devillers, M., Sirch, M. & Penzhorn, R. D. Hydrogen-induced disproportionation of the intermetallic zirconium-cobalt compound ZrCo. Chem. Mater. 4, 631–639 (1992).
-
Naik, Y., Rama Rao, G. A. & Venugopal, V. Zirconium–cobalt intermetallic compound for storage and recovery of hydrogen isotopes. Intermetallics 9, 309–312 (2001).
-
Jat, R. A., Parida, S. C., Nuwad, J., Agarwal, R. & Kulkarni, S. G. Hydrogen sorption–desorption studies on ZrCo–hydrogen system. J. Therm. Anal. Calorim. 112, 37–43 (2013).
-
Devillers, M., Sirch, M., Bredendiekkamper, S. & Penzhorn, R. D. Characterization of the ZrCo-hydrogen system in view of its use for tritium storage. Chem. Mater. 2, 255–262 (1990).
-
Hayashi, T. et al. Development of ZrCo beds for ITER tritium storage and delivery. Fusion Sci. Technol. 41, 801–804 (2002).
-
Huang, X. et al. Kinetic hydrogen isotope effects and flow compensation strategy of the ZrCo-based chemical beds in full-scale SDS demo-system. Fusion Eng. Des. 192, 113748 (2023).
-
Shim, M. et al. Experimental study on the delivery rate and recovery rate of ZrCo hydride for ITER application. Fusion Sci. Technol. 54, 27–30 (2008).
-
Beloglazov, S., Glugla, M., Wagner, R., Fanghänel, E. & Grünhagen, S. Investigation of isotope effects in the gas streams supplied by a 1:1 ITER storage bed using a micro gas chromatography. Fusion Sci. Technol. 48, 67–70 (2005).
-
Jat, R., Rawat, D. & Sharma, A. Isotope effect on hydrogen storage properties of Ti and Nb co-substituted ZrCo alloy. Int. J. Hydrogen Energy 48, 16802–16812 (2023).
-
Yan, Q. et al. A squarate-pillared titanium oxide quantum sieve towards practical hydrogen isotope separation. Nat. Commun. 14, 4189 (2023).
-
Hayashi, T., Suzuki, T., Shu, W. M. & Yamanishi, T. Isotope effect of hydrogen rapidly supplied from the metal storage bed. Fusion Sci. Technol. 52, 706–710 (2007).
-
Yang, Y. et al. Isotope effects in Ti0.3V0.4Cr0.3–hydrogen system: The dependence of αH−D on pressure, deuterium concentration and temperature. J. Alloy. Compd. 683, 15–21 (2016).
-
Yang, Y., Luo, D., Guo, W. & Ye, X. Hydrogen isotope effects in Ti–V–Cr alloy hydrides. J. Phys. Chem. C. 119, 3481–3487 (2015).
-
Yuan, Y. et al. Honeycomb ZrCo intermetallic for high performance hydrogen and hydrogen isotope storage. ACS Appl. Mater. Interfaces 15, 3904–3911 (2023).
-
Liang, Z. et al. A new strategy for remarkably improving anti-disproportionation performance and cycling stabilities of ZrCo-based hydrogen isotope storage alloys by Cu substitution and controlling cutoff desorption pressure. Int. J. Hydrog. Energy 44, 28242–28251 (2019).
-
Liang, Z. et al. Regulating local chemistry in ZrCo-based orthorhombic hydrides via increasing atomic interference for ultra-stable hydrogen isotopes storage. J. Energy Chem. 69, 397–405 (2022).
-
Liang, Z. et al. The functioning mechanism of Al valid substitution for Co in improving the cycling performance of Zr–Co–Al based hydrogen isotope storage alloys. J. Alloy. Compd. 848, 156618 (2020).
-
Liang, Z. et al. Dual-ion substitution-induced unique electronic modulation to stabilize an orthorhombic lattice towards reversible hydrogen isotope storage. ACS Sustain. Chem. Eng. 9, 9139–9148 (2021).
-
Liang, Z. et al. Positive impacts of tuning lattice on cyclic performance in ZrCo-based hydrogen isotope storage alloys. Mater. Today Energy 20, 100645 (2021).
-
Yao, Z. et al. An impact of hydrogenation phase transformation mechanism on the cyclic stabilizing behavior of Zr0.8Ti0.2Co alloy for hydrogen isotope handling. Mater. Today Energy 18, 100554 (2020).
-
Yao, Z. et al. Achieving excellent cycle stability in Zr–Nb–Co–Ni based hydrogen isotope storage alloys by controllable phase transformation reaction. Renew. Energy 187, 500–507 (2022).
-
Yao, Z. et al. Improvement on the kinetic and thermodynamic characteristics of Zr1-xNbxCo (x = 0–0.2) alloys for hydrogen isotope storage and delivery. J. Alloy. Compd. 784, 1062–1070 (2019).
-
Kou, H. et al. Effects of ball milling on hydrogen sorption properties and microstructure of ZrCo alloy. Fusion Eng. Des. 138, 68–77 (2019).
-
Qi, J. et al. Effect of isostructural phase transition on cycling stability of ZrCo-based alloys for hydrogen isotopes storage. Chem. Eng. J. 455, 140571 (2023).
-
Zhang, G. et al. Effects and mechanism of Ti, Ni, Sc, Fe substitution on the thermal stability of zirconium cobalt–hydrogen system. Int. J. Hydrog. Energy 40, 6582–6593 (2015).
-
Huang, G. et al. Spatial-confinement synthesis of single-crystal ZrCo nanoparticles for ultrafast and long-life hydrogen/hydrogen isotope storage. Chem. Eng. J. 473, 145342 (2023).
-
Jat, R. A., Parida, S. C., Agarwal, R. & Ramakumar, K. L. Investigation of hydrogen isotope effect on storage properties of Zr–Co–Ni alloys. Int. J. Hydrog. Energy 39, 14868–14873 (2014).
-
Jat, R. A., Parida, S. C., Agarwal, R. & Kulkarni, S. G. Effect of Ni content on the hydrogen storage behavior of ZrCo1-xNix alloys. Int. J. Hydrog. Energy 38, 1490–1500 (2013).
-
Jat, R. A. et al. Structural and hydrogen isotope storage properties of Zr–Co–Fe alloy. Int. J. Hydrog. Energy 40, 5135–5143 (2015).
-
Jat, R. A., Pati, S., Parida, S. C., Agarwal, R. & Mukerjee, S. K. Synthesis, characterization and hydrogen isotope storage properties of Zr–Ti–Co ternary alloys. Int. J. Hydrog. Energy 42, 2248–2256 (2017).
-
Monea, B. F. et al. Synthesis, characterization, and hydrogen isotope storage properties of Zr1-xTixCo and Zr1-xHfxCo alloys (x = 0.1, 0.2). Fusion Sci. Technol. 77, 382–390 (2021).
-
Jat, R. A., Rawat, D., Sharma, A. & Parida, S. C. Remarkable enhancement in durability of ZrCo alloy against hydrogen induced disproportionation by Ti and Nb co-substitution. Int. J. Hydrog. Energy 48, 7431–7441 (2023).
-
Chattaraj, D., Kumar, N., Ghosh, P., Majumder, C. & Dash, S. Adsorption, dissociation and diffusion of hydrogen on the ZrCo surface and subsurface: A comprehensive study using first principles approach. Appl. Surf. Sci. 422, 394–405 (2017).
-
Zhang, B., Ye, X., Luo, W. & Sang, G. Influence of niobium/tantalum doping on the hydrogen behavior of ZrCo(110) surface. Int. J. Hydrog. Energy 48, 17577–17592 (2023).
-
Wang, W. et al. Temperature dependence of hydrogen isotope effect in LaNi5-H(D) system. Nucl. Anal. 2, 100051 (2023).
-
Falin, A. et al. Anomalous isotope effect on mechanical properties of single atomic layer boron nitride. Nat. Commun. 14, 5331 (2023).
-
Giles, A. J. et al. Ultralow-loss polaritons in isotopically pure boron nitride. Nat. Mater. 17, 134–139 (2018).
-
Lundin, C. E., Lynch, F. E. & Magee, C. B. A correlation between the interstitial hole sizes in intermetallic compounds and the thermodynamic properties of the hydrides formed from those compounds. J. Less Common Met. 56, 19–37 (1977).
-
Lewis, F. A. Hydrogen isotope absorption and separation by palladium and palladium alloys. J. Less Common Met. 74, 363–369 (1980).
-
Du, X., Ye, X., Chen, C., & Jiang, C. Hydrogen isotope effect of nanoporous palladium. Int. J. Hydrog. Energy 46, 1023–1029 (2021).
-
Glugla, M., Cristescu, I. R., Cristescu, I. & Demange, D. Hydrogen isotope separation by permeation through palladium membranes. J. Nucl. Mater. 355, 47–53 (2006).
-
Nishikawa, M., Shiraishi, T. & Murakami, K. Solubility and separation factor of protium-deuterium binary component system in palladium. J. Nucl. Sci. Technol. 33, 504–510 (1996).
-
Wang, L. & Yang, R. T. New sorbents for hydrogen storage by hydrogen spillover – a review. Energy Environ. Sci. 1, 268–279 (2008).
Acknowledgements
We are grateful for the financial supports from the National Natural Science Foundation of China (52071286 and U2030208) and the National Key Research and Development Program of China (2022YFE03170002). We thank Dr. Zhemin Wu for the HRTEM imaging and analyzing.
Ethics declarations
Competing interests
The authors declare no competing interests.
Peer review
Peer review information
Nature Communications thanks Silvano Tosti, Suresh Parida and the other, anonymous, reviewer for their contribution to the peer review of this work. A peer review file is available.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.